Addario, N., Rizzuto, E., & Prestileo, A. (2014). Ship Side Damage Due to Collision with Icebergs. In: Soares G, Pena L, eds.
Developments in Maritime Transportation and Exploitation of Sea Resources. London. Taylor & Francis Group.

Atlantic Survey. (1982). Iceberg Aerial Reconnaissance, Atlantic Survey Report for Mobil Hibernia Development Studies.
Bailey, J., Marino, A., & Akbari, V. (2021). Comparison of Target Detectors to Identify Icebergs in Quad-polarimetric L-band.
Synthetic Aperture Radar Data Remote Sensing,
13(9), 1753.
https://doi.org/10.3390/rs13091753

Barbat, MM., Wesche, C., Werhli, AV., & Mata, MM. (2019). An Adaptive Machine Learning Approach to Improve Automatic Iceberg Detection From SAR Images.
Journal of Photogrammetry and Remote Sensing,
156, 247-259.
https://doi.org/10.1016/j.isprsjprs.2019.08.015

Bercha, FG. (1983). Iceberg Aerial Reconnaissance 1983. F.G. Bercha and Associates Report for Mobil Hibernia Development Studies.
Bercha, FG. (1984). Iceberg Aerial Reconnaissance 1984. F.G. Bercha and Associates Report for Mobil Hibernia Development Studies.
Cammaert, AB., & Muggeridge, DB. (1988). Ice Interaction with Offshore Structures. New York. Van Nostrand Reinhold.
Choi, J. (2018). Iceberg-ship Classification in SAR Images Using Convolutional Neural Network with Transfer Learning.
Journal of Internet Computing and Services,
19(4), 35-44.
https://doi.org/10.7472/jksii.2018.19.4.35

Choi, K. (2013). Sea Ice in Polar Regions. Seoul, Korea. GeoBook Publishing Co.
Daley, C., & Veitch, B. (2000).
Iceberg Evolution Modeling: A Background Study (PERD/CHC Report, OERC Report 2000-05-06). National Research Council of Canada:
https://doi.org/10.4224/12341002

Dobrocky Seatech. (1984). Iceberg Field Survey 1984. Mobil Hibernia Development Studies.
Dobrocky Seatech. (1985). Iceberg Dimensions and Shape Field Survey 1985, Terra Nova Development Studies.
Fenco. (1971). Iceberg Dynamics Project, Report by Fenco (Newfoundland) Ltd.
Foumler, M., Lasserre, F., Beveridge, L., & Tetu, PL. (2020). A European Shipping Companies Survey on Arctic Shipping: Expectation vs. Reality. Quebec Council for Geopolitical Studies (CQEG). Retrieved from
http://hdl.handle.net/20.500.11794/39514
Gammon, PH., Gagnon, RE., Bobby, W., & Russell, WE. (1983). Physical and Mechanical Properties of Icebergs.
Proceedings of the Offhsore Technology Conference. Houston, Texas. p 143-150.
https://doi.org/10.4043/4459-MS

Haynes, FD. (1978). Effect of Temperature on the Strength of Snow-ice (CRREL Report 78‒27). Hanover, New Hampshire: Department of the Army, Cold Regions Research and Engineering Laboratory, Corps of Engineers.
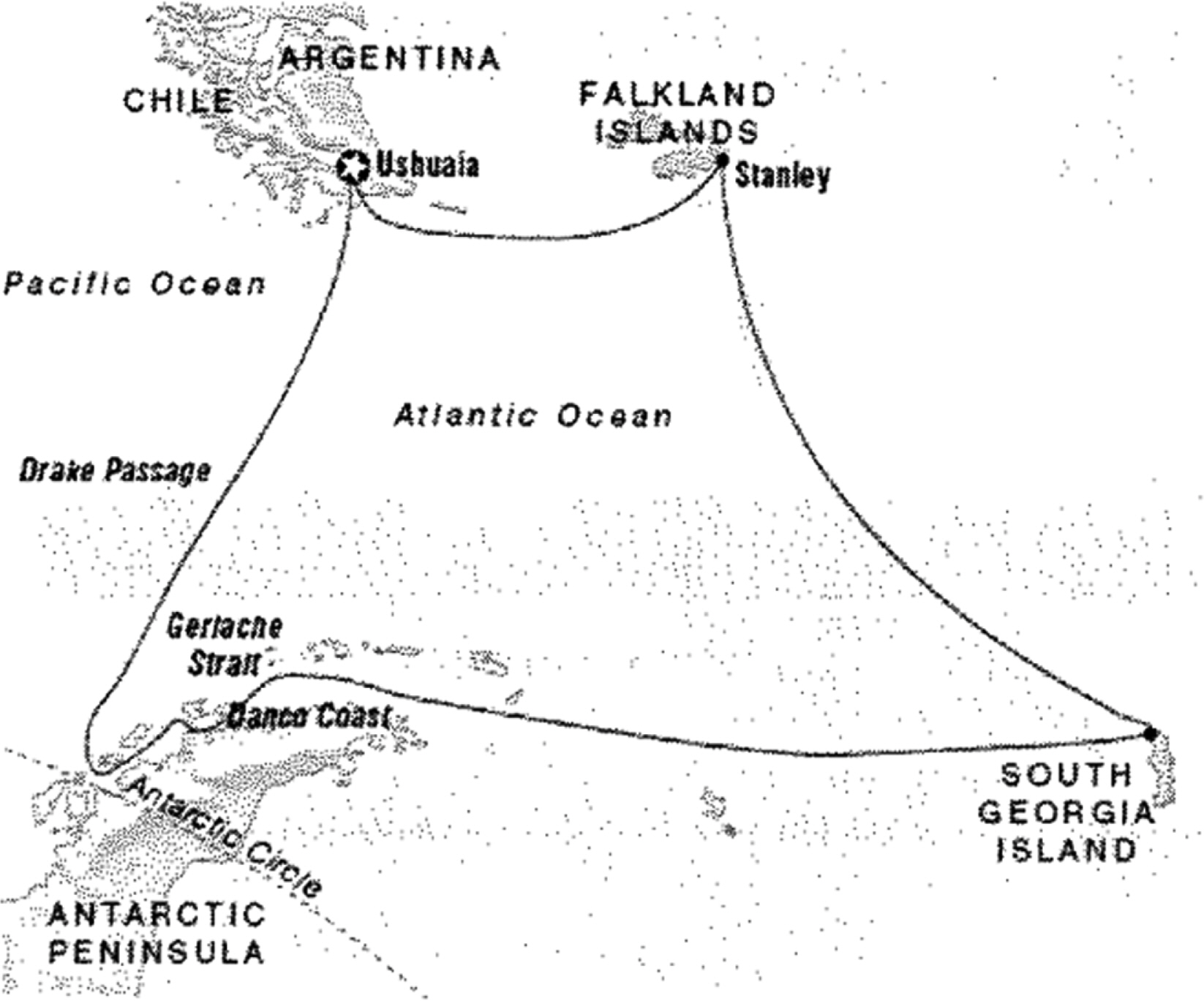
Hill, B. (2001). Ship Collisions with Icebergs: an Historical Record of Collisions in the Seas Around North America and Greenland. Proceedings of the 16th International Conference on Port and Ocean Engineering Under Arctic Conditions. Ottawa, Canada. 2: p 997-1002.
Hodgson, GJ., Lever, JH., Woodworth-Lynas, CM., & Lewis, CF. (1988). The Dynamics of Iceberg Grounding and Scouring (DIGS) Experiment and Repetitive Mapping of the Eastern Canadian Cntinental Self (ESRF Report 09). Ottawa. Environmental Studies Research Funds.
Høyland, KV., Kim, E., Marchenko, A., Lishman, B., & Barrette, P. (2020). Ice Properties in ISO 19906’s. 2nd ed Proceedings of 25th IAHR International Symposium on Ice. Trondheim, Virtual Conference.
ICE Engineering Ltd. (1983a). Iceberg Field Survey, Ice Engineering Report for Mobil Hibernia Development Studies.
ICE Engineering Ltd. (1983b). A Survey of Icebergs in the Strait of Belle Isle, Ice Engineering Report to Bedford Institute of Oceanography.
ICE Engineering Ltd. (1984). Iceberg Survey – Grand Banks – Hibernia, Contract Report Prepared for BIO.
ICE Engineering Ltd. (1985). Iceberg Survey – Grand Banks – Hibernia, Contract Report Prepared for BIO.
Intera. (1981). Iceberg Dimensions by Aerial Photography, Intera Environmental Consultants Ltd., Report for Mobile Hibernia Development Studies.
International Standards Organization. (2019). Petroleum and Natural Gas Industries — Arctic Offshore Structures (ISO 19906).
Jang, KB., Che, JS., & Choo, IH. (1997). Iceberg Collision Simulation for Ice Class Shuttle Tanker, Samsung Internal Technical Report M37 KR 96001..
Jia, Z., Ringsberg, JW., & Jia, J. (2009). Numerical Analysis of Nonlinear Dynamic Structural Behaviour of Ice-loaded Side-shell Structures.
International Journal of Steel Structures,
9, 219-230.
https://doi.org/10.1007/BF03249496

Kim, JH. (2007). A Study on Standardization of Ice Mechanics Experimental Techniques with a Cold Room (Master thesis). Korea Maritime University.
Korzhavin, KN. (2002). Action of Ice on Engineering Structures. New York. Books for Business. (Original work published 1971).
Lee, RW., & Schulson, EM. (1988). The Strength and Ductility of Ice Under Tension.
Journal of Offshore Mechanics and Arctic Engineering,
110(2), 187-191.
https://doi.org/10.1115/1.3257049

Marchenko, N. (2012). Russian Arctic Seas: Navigational Conditions and Accidents. Heidelberg. Springer Science & Business Media..
Marchenko, N. (2014). Floating Ice Induced Ship Casualties. Proceeding of 22nd IAHR International Symposium on Ice. Singapore.
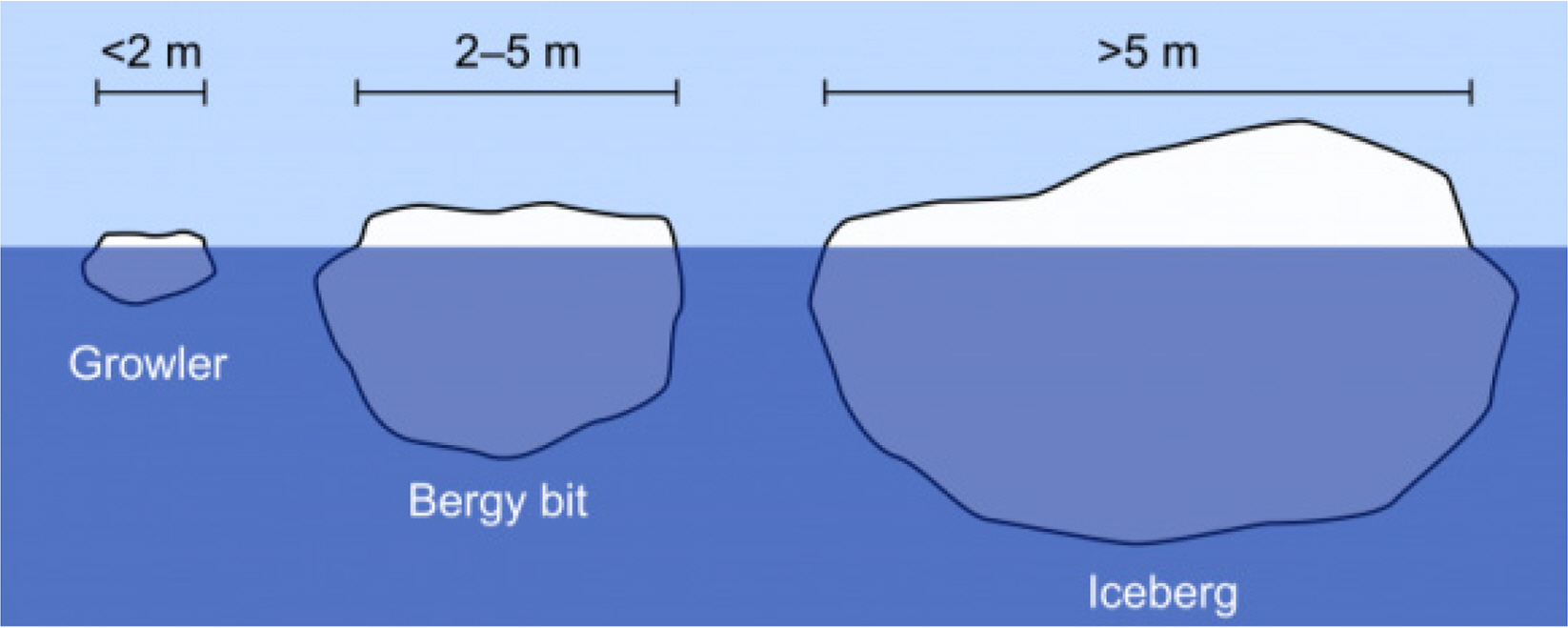
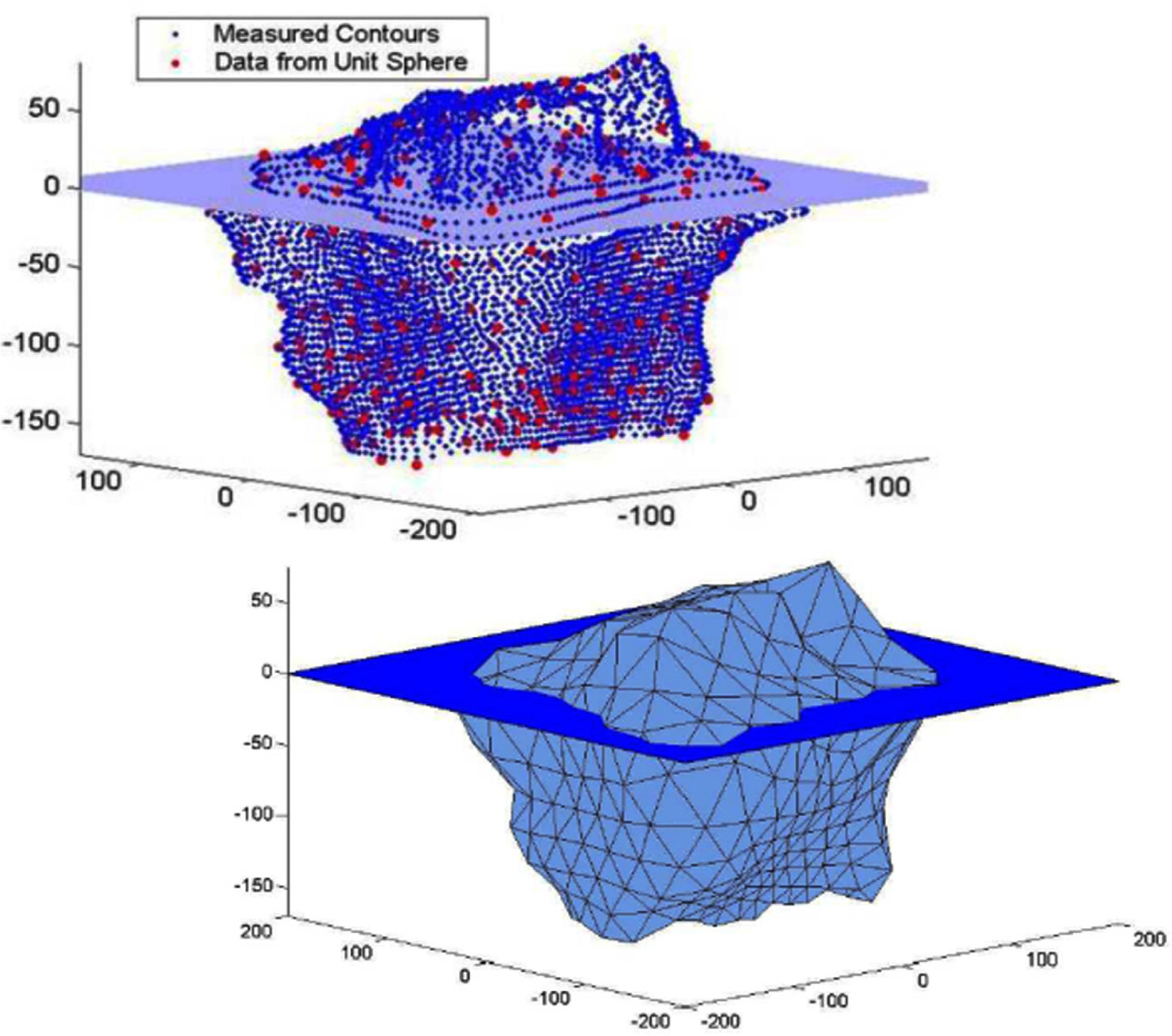
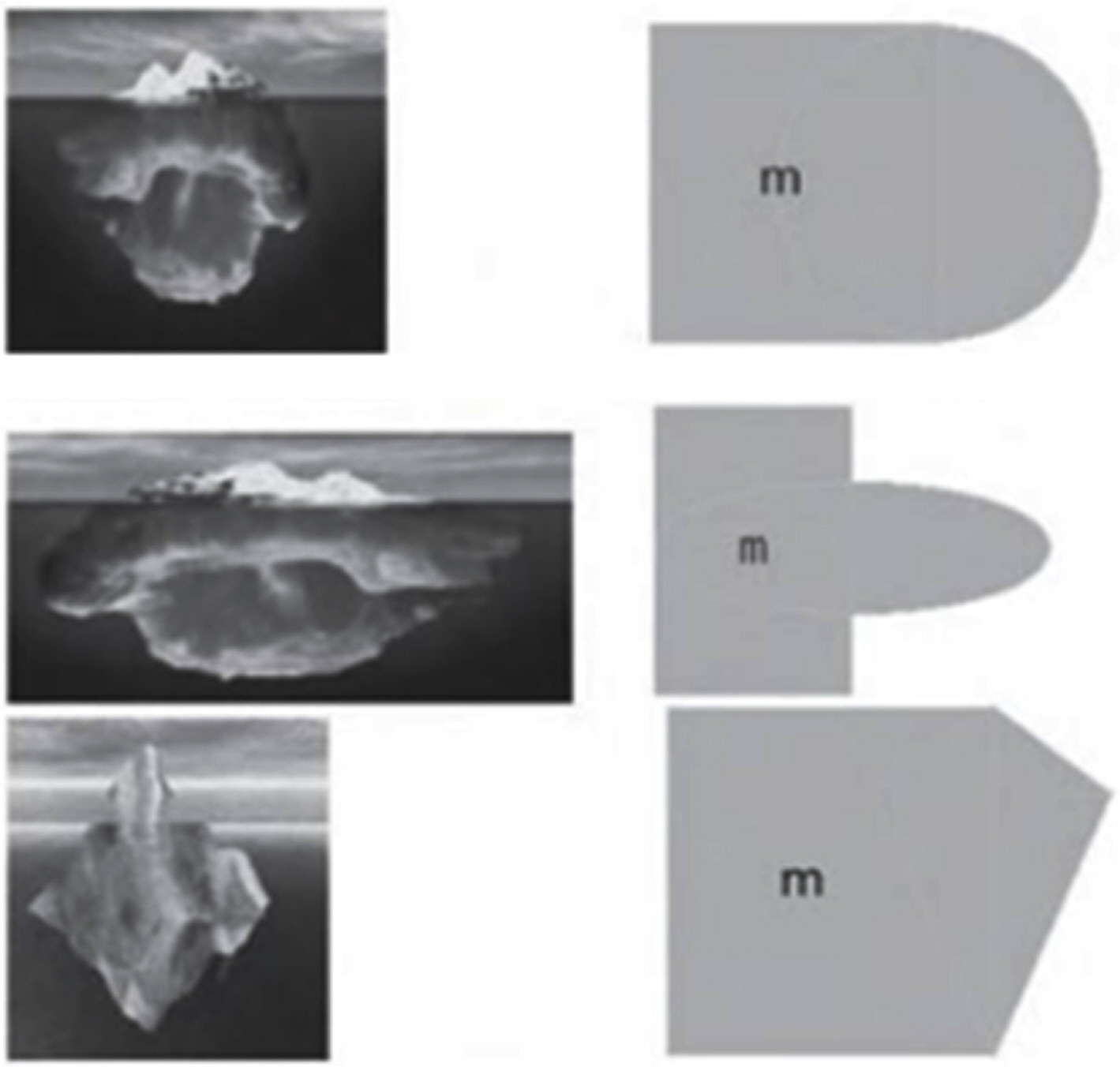
McKenna, RF. (2004). Development of Iceberg Shape Characterization for Risk to Grand Banks Installations (PERD/CHC Report 20-73).
https://doi.org/10.4224/12340897

McKenna, RF. (2005). Iceberg Shape Charaterization. Proceedings 18th International Conference on Port and Ocean Engineering under Arctic Conditions, 2, 555-564.
Mejlaelander-Larsen, M., & Hysing, T. (2006). Ice Collision Scenario (Technical Report 2006-0672). DNV.
Oceans Ltd. (2004). Determination of Iceberg Draft and Shape (PERD/CHC Report), Oceans Ltd.
Paik, JK. (1992). Lessons from Grounding Accident of Exxon Vadez. Bullutin of Society of Naval Architects of Korea, 29(2), 27-31.
Palmer, A., & Croasdale, K. (2013). Arctic Offshore Engineering. Singapore. World Scientific Publishing.
Paterson, WSB. (1994). The Physics of Glaciers. 3rd ed Oxford. Elsevier Science Ltd.
Sanderson, TJO. (1988). Ice Mechanics: Risks to Offshore Structures. London. Graham & Trotman.
Smith, SD., & Donaldson, NR. (1987). Dynamic Modelling of Iceberg Drift Using Current Profiles. Canadian Technical Report of Hydrography and Ocean Sciences 91. Bedford Institute of Oceanography.
Soldal, I., Dierking, W., Korosov, A., & Marino, A. (2019).
Automatic Detection of Small Icebergs in Fast Ice Using Satellite Wide-swath SAR Images, Remote Sensing,
11(7), 806.
https://doi.org/10.3390/rs11070806

Suh, Y., Ito, H., Chun, S., Han, S., Choi, J., & Urm, H. (2008). Ice Collision Analysis for Membrane Tank Type LNG Carrier. Journal of Ship & Ocean Technology, 12(1), 35-44.
Turnbull, ID., Fournier, N., Stolwijk, M., Fosnaes, T., & McGonigal, D. (2015). Operational Iceberg Drift Forecasting in Northwest Greenland.
Cold Regions Science and Technology,
110, 1-18.
https://doi.org/10/1016/j.coldregions.2014.10.006

US Army Corps of Engineers. (2005). Engineering and Design: Ice Engineering, Honolulu, Hawaii USA. University Press of the Pacific. (Original work published 2002).
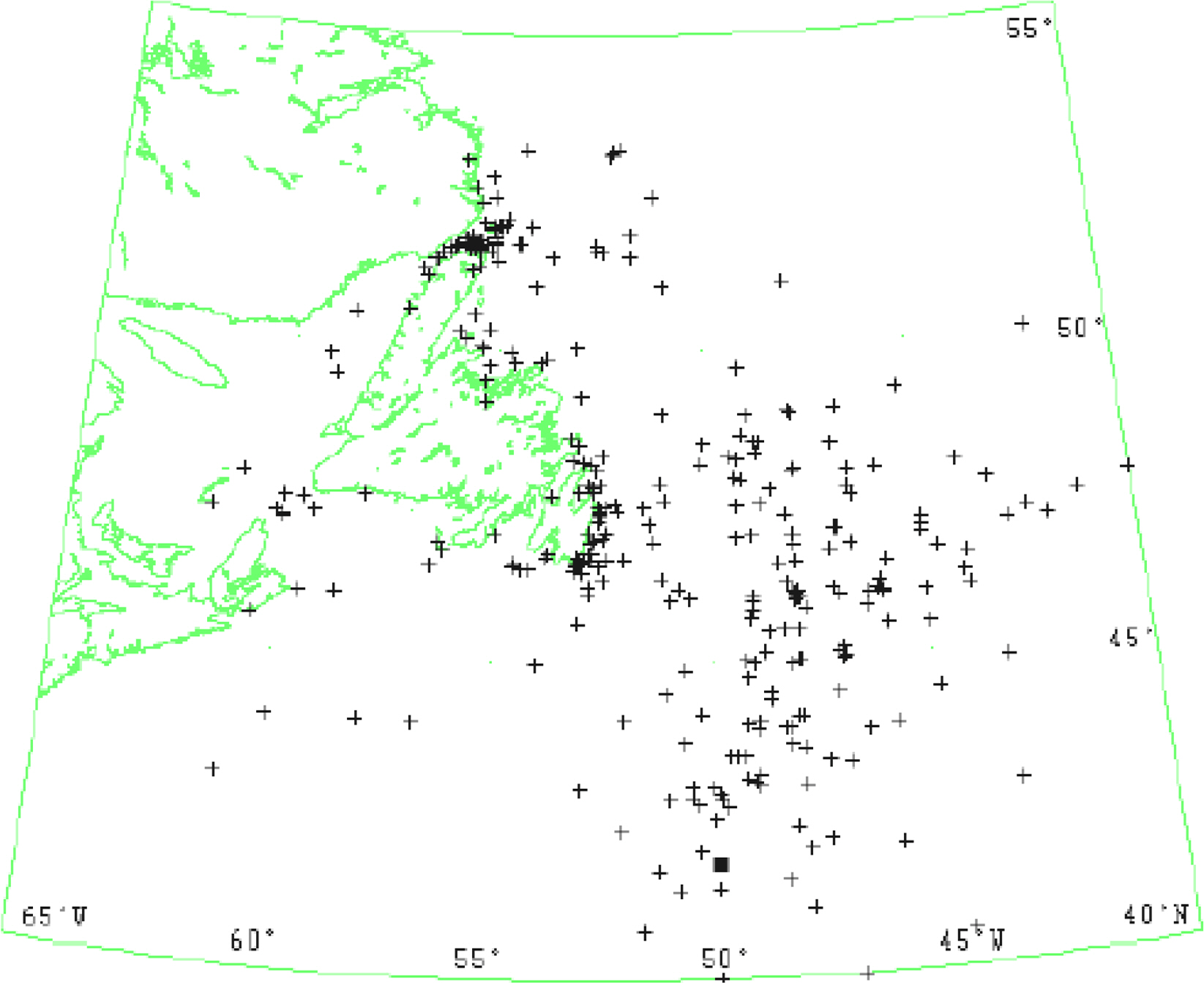
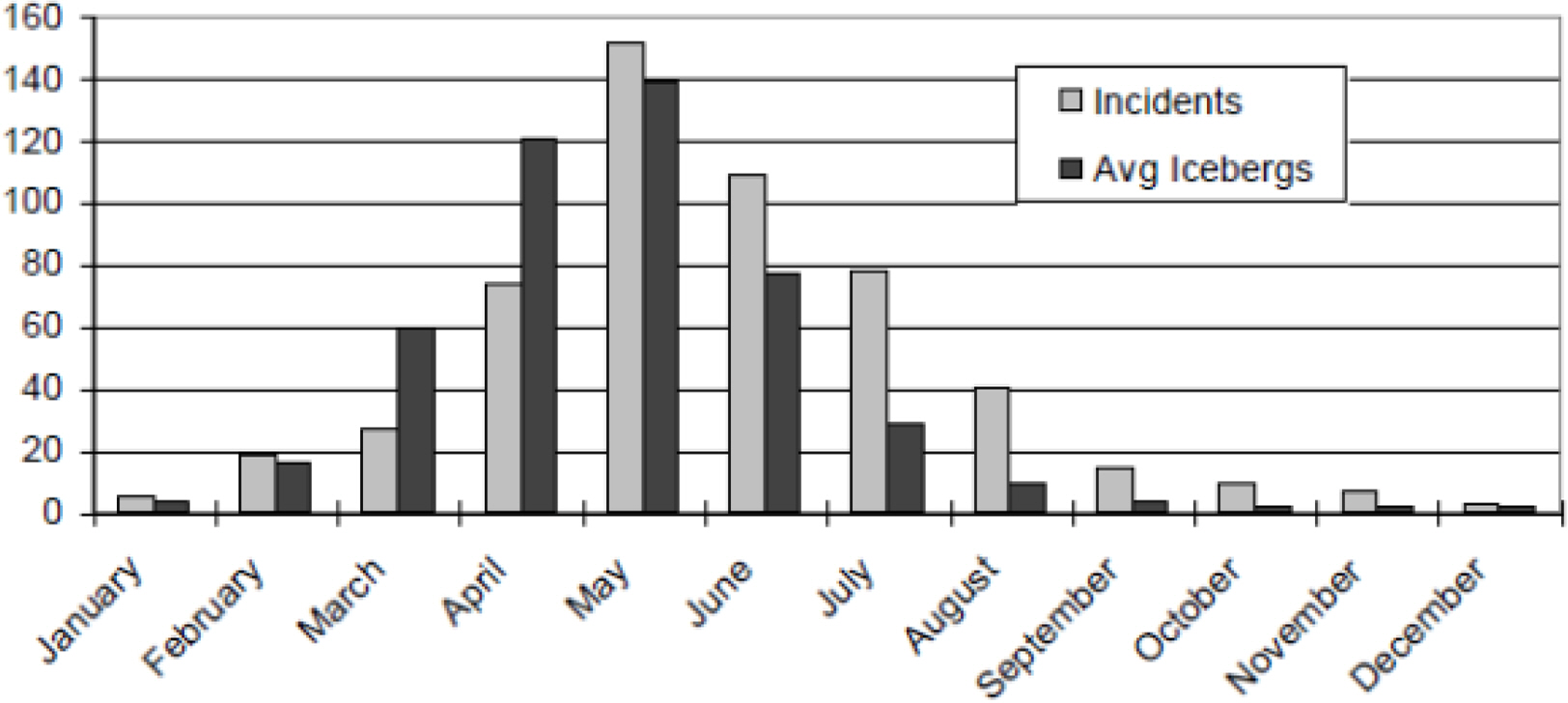
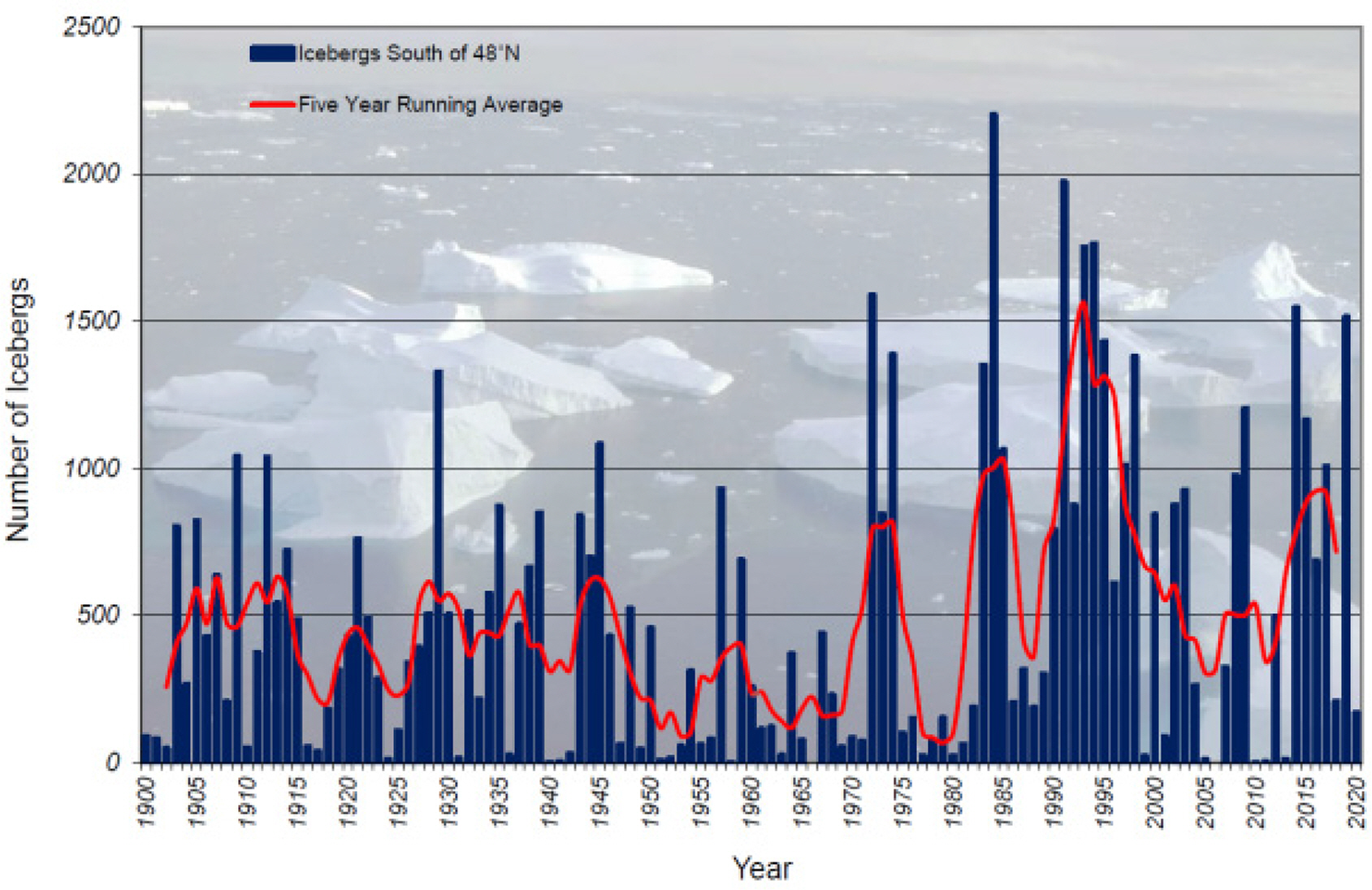
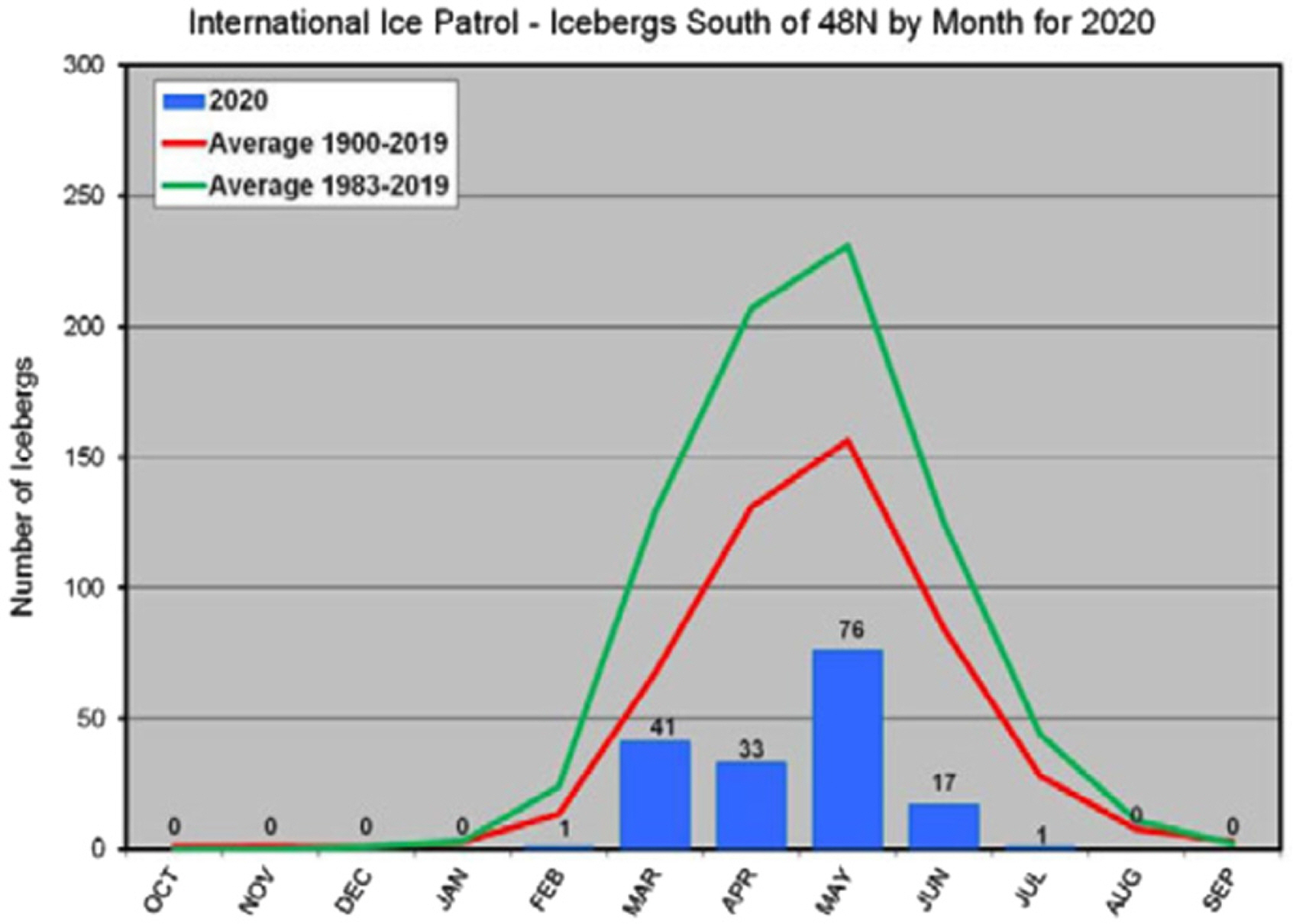
USCG. (2020). Report of the International Ice Patrol in the North Atlantic, 2020 Season Bulletin, 106, CG-188-75, US Homeland Security, US Coast Guard.