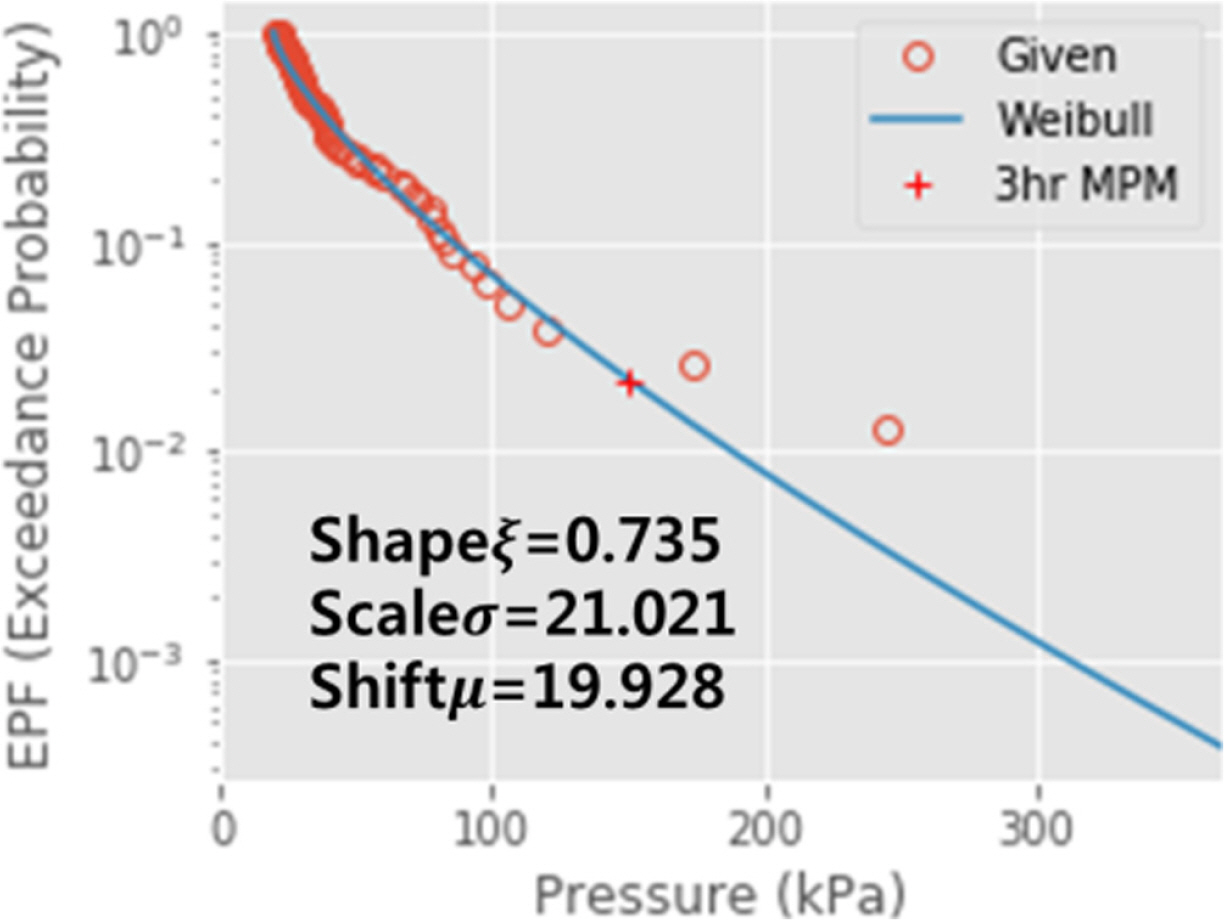
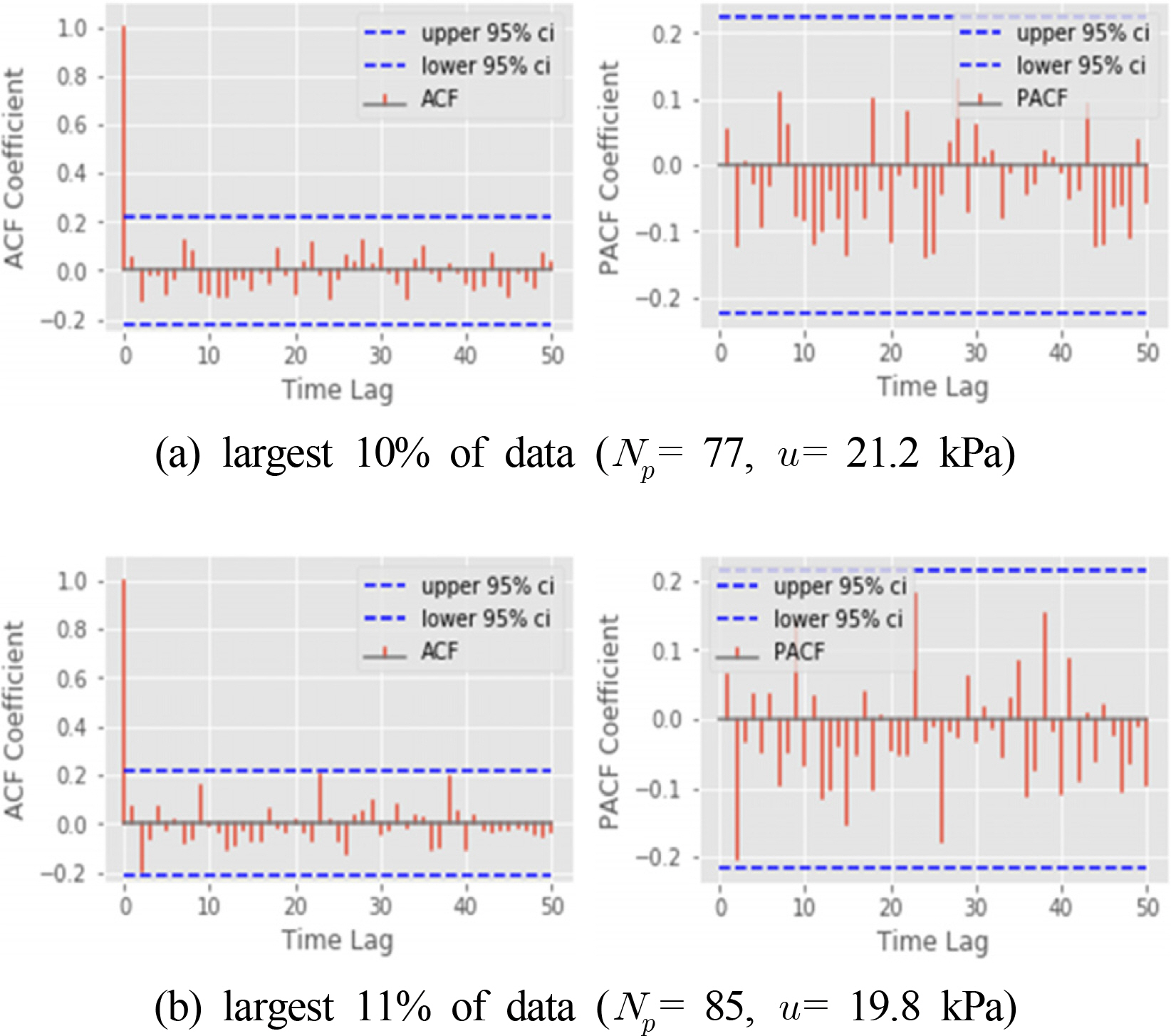
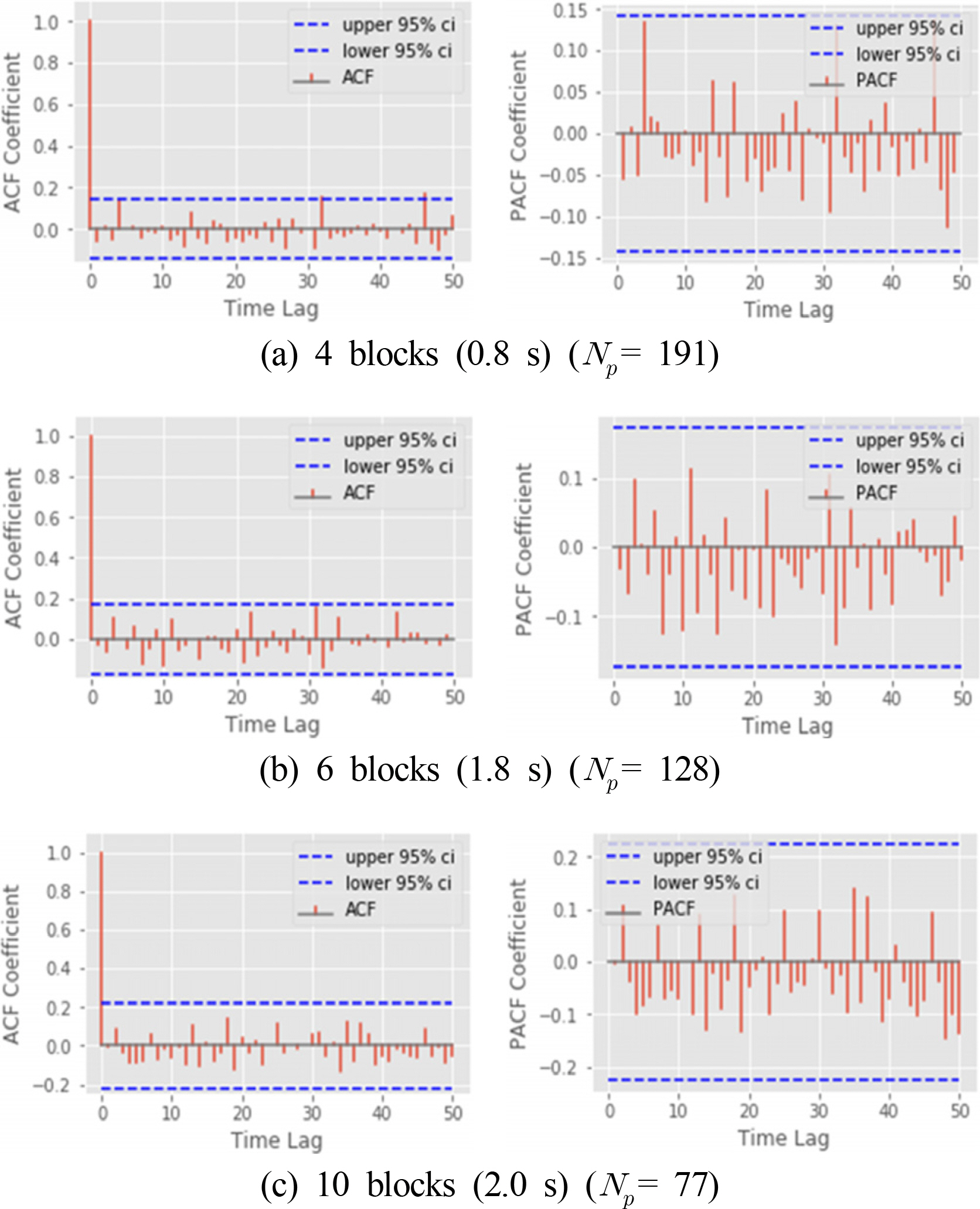
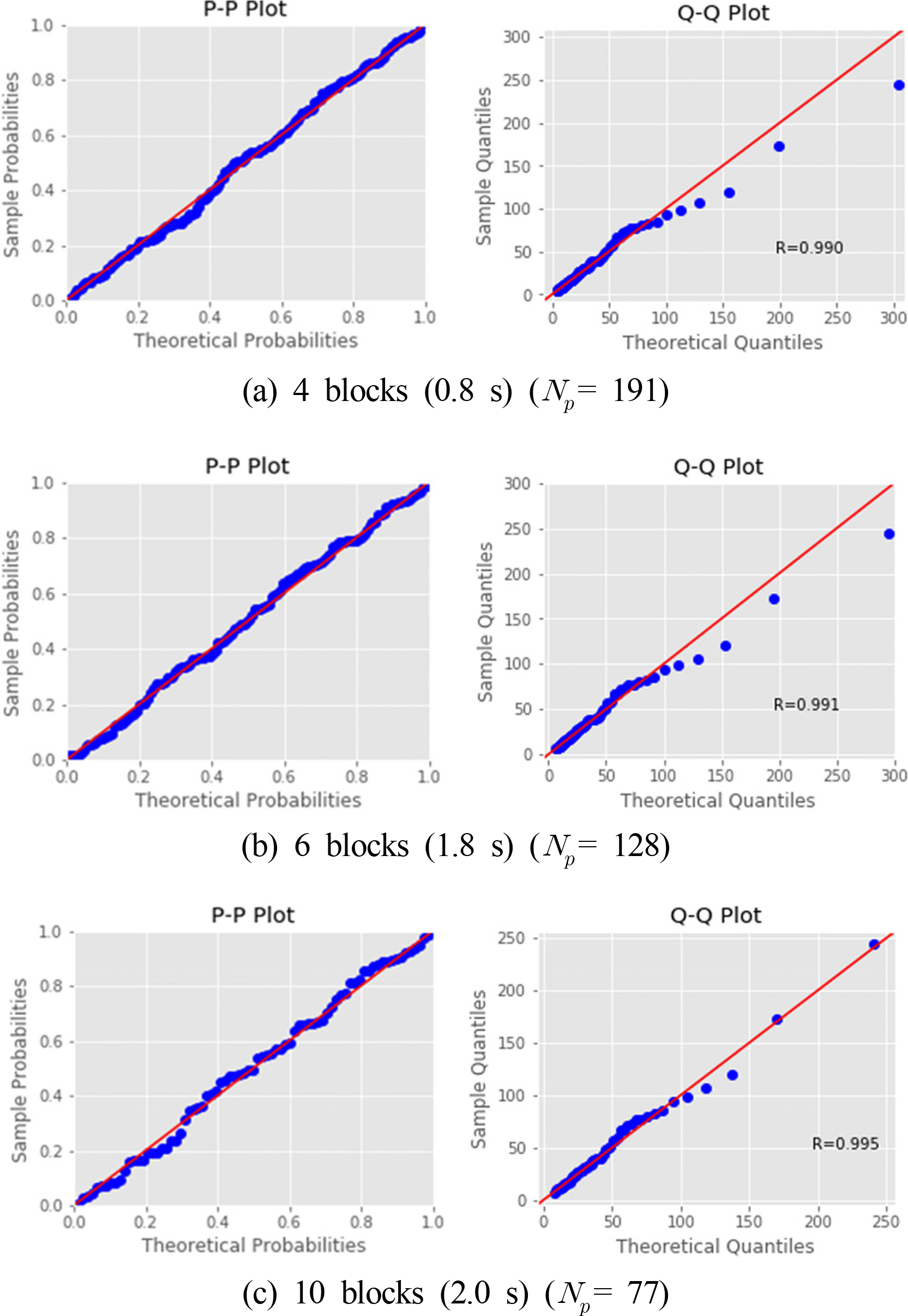
Balkema, A., & de Haan, L. (1974). Residual Life Time at Great Age.
The Annals of Probability,
2(5), 792-804.

Bommier, E. (2014). Peaks-over-threshold Modeling of Environmental Data. Project Report, 2014, 33. Department of Mathematics, Uppsala University. DiVA, id: diva2:760802..
Choi, YH. (2016). Extreme Value Analysis of Environmental Data. Proceedings of the Fall Conference of the Korean Society of Ocean Engineers, 181-186.
DNVGL. (2015). Position Mooring. DNVGL-OS-E301..
DNVGL. (2017). Environmental Conditions and Environmental loads. DNVGL-RP-C205..
Fillon, B., Diebold, L., Henry, J., Derbanne, Q., Baudin, E., & Parmentier, G. (2011). Statistical Post-Processing of Long-Duration Sloshing Test. ISOPE-I-11-526.
Fisher, RA., & Tippett, LHC. (1928). Limiting Forms of the Frequency Distribution of the Largest and Smallest Member of a Sample.
Mathematical Proceedings of the Cambridge Philosophical Society,
24(2), 180-190.

Gnedenko, BV. (1943). Sur La Distribution Limite Du Terme Maximum DŌĆÖUne S├®rie Al├®atoire.
Annals of Mathematics,
44(3), 423-453.

Gumbel, EJ. (1954). Statistics of Extremes. Dover: Mineola, New York. ISBN 0-486-43604-7..
Smith, RL. (1985). Maximum Likelihood Estimation in a Class of Nonregular Cases.
Biometrika,
72(1), 67-90.

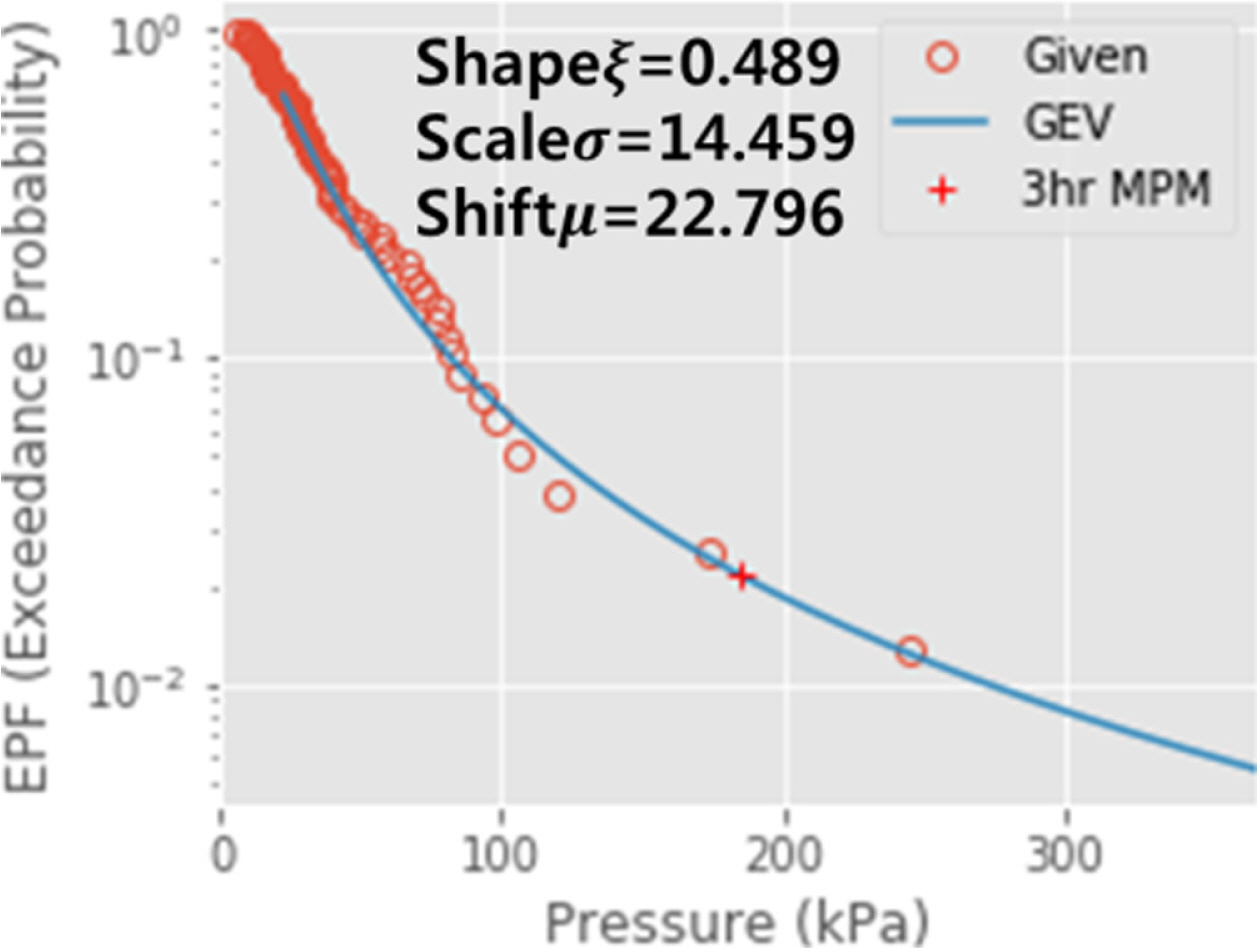
Kim, S. (2017). Development of Long-term Prediction Procedure and Outlier Analysis for Sloshing Impact Loads on LNG Cargo Tank. Doctoral Thesis Seoul National University.
ITTC. (2017). ITTC Quality System Manual - Recommended Procedures and Guidelines - Procedure - Sloshing Model Tests. 7.5-02-07-02.7.
Ochi, MK. (1990). Applied Probability and Stochastic Processes ŌĆō In Engineering and Physical Sciences. John Wiley & Sons: ISBN-10: 0471857424, ISBN-13: 9780471857426.
Pickands, J. (1975). Statistical Inference Using Extreme Order Statistics.
The Annals of Statistics,
3(1), 119-131.

Tukey, JW. (1977). Exploratory Data Analysis. Addison-Wesley Publishing Company: 1st Edition ISBN-10: 0201076160, ISBN-13: 9780201076165.
Xu, J., & Long, JS. (2005). Using the Delta Method to Construct Confidence Intervals for Predicted Probabilities, Rates, and Discrete Changes Lecture Notes. Indiana University.